In mathematics, the lowest common denominator or least common denominator is the lowest common multiple of the denominators of a set of fractions. It simplifies adding, subtracting, and comparing fractions. The lowest common denominator or least common denominator is the least common multiple of the denominators of a set of fractions. LCD is the smallest positive integer that is multiple denominators in the set. Fractions write with fraction bar / like 3/4 .
In this example, the least common multiple of 3 and 6 must be determined. In other words, "What is the smallest number that both 3 and 6 can divide into evenly? " With a little thought, we realize that 6 is the least common multiple, because 6 divided by 3 is 2 and 6 divided by 6 is 1. The fraction \(\frac\) is then adjusted to the equivalent fraction \(\frac\) by multiplying both the numerator and denominator by 2.
Now the two fractions with common denominators can be added for a final value of \(\frac\). Taking out the LCM is a helpful skill when adding or subtracting fractions. Determining the lowest common multiple creates a denominator that is the same for both fractions. For example, the common denominator for \(\frac+\frac\) would be \(35\), because \(35\) is the LCM of \(7\) and \(5\). The new fractions become \(\frac+\frac\), which equals \(\frac\).
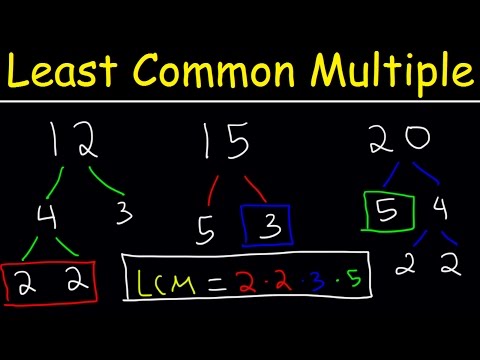
As that last example illustrates, if two numbers have no prime factors in common, the lowest common multiple will be equal to the product of the two numbers. The other way to find the lowest common multiple is to list the prime factors for each number. Remove the prime factors both numbers have in common. Multiply one of the numbers by the remaining prime factors of the other number. The result will be the lowest common multiple.
We can see 6 and 12 are included in both rows. Therefore, they are multiples of both 2 and 3. However, the smaller of the two is 6, and it is called the least common multiple of 2 and 3. Then we can write 1/2 and 1/3 as equivalent fractions with 6 in the denominator.
This allows addition and subtraction to be performed on the two fractions easily. Denominator is the lower part of a vulgar fraction. I.e. a fraction given in the form a/b , where b is the denominator. A common denominator is a common multiple of all the denominators of two or more vulgar fractions. Specifically, the lowest common denominator or least common denominator is the important one. Least common multiple of all the denominators is known as the lowest common denominator.
To find common denominator or to find the least common denominator there are several methods. Similarly, when we add fractions with different denominators we have to convert them to equivalent fractions with a common denominator. With the coins, when we convert to cents, the denominator is 100[/latex]. Since there are 100[/latex] cents in one dollar, 25[/latex] cents is \frac[/latex] and 10[/latex] cents is \frac[/latex]. So we add \frac+\frac[/latex] to get \frac[/latex], which is 35[/latex] cents.
Now that methods for finding least common multiples have been introduced, we'll need to change our mindset to finding the greatest common factor of two or more numbers. We will be identifying a value smaller than or equal to the numbers being considered. In other words, ask yourself, "What is the largest value that divides both of these numbers? " Understanding this concept is essential for dividing and factoring polynomials.
If one number is prime, and the other number's prime factors include that prime number, the lowest common multiple will be equal to the non-prime number. Implement any of the methods used for finding the LCD of common fractions, as explained in the previous method sections. The least common denominator, also known as the lowest common denominator, is the lowest common multiple of the denominators of a given set. When finding the GCF, start by listing the prime factorization of each number . For example, the prime factorization of \(45\) is \(5\times3\times3\), and the prime factorization of \(120\) is \(5\times3\times2\times2\times2\).
Now simply multiply all of the factors that are shared by both numbers. In this case, we would multiply \(5\times3\) which equals \(15\). Prime factorization can also be used to determine the greatest common factor. However, rather than multiplying all the prime factors like we did for the least common multiple, we will multiply only the prime factors that the numbers share.
The resulting product is the greatest common factor. One way to do this is to write out all of the multiples of both denominators, then see where they match for the first time. You can also factor both the denominators and see if there are any common factors. If they do share common factors, the ones they do not have in common will give you insight into how to get the least common denominator. Scan through each list and mark any multiples that are shared by all of the original denominators. After identifying the common multiples, identify the lowest multiple common to all the denominators.
The least common denominator is the smallest common denominator. It is the smallest whole number that is evenly divisible by all uncommon denominators. The least common denominator is also referred to as the lowest common denominator or the least common multiple. To add or subtract fractions with different denominators, we will first have to convert each fraction to an equivalent fraction with the LCD. Let's see how to change \frac\text\frac[/latex] to equivalent fractions with denominator 12[/latex] without using models.
The lcm is the "lowest common denominator" that can be used before fractions can be added, subtracted or compared. Since division of integers by zero is undefined, this definition has meaning only if a and b are both different from zero. However, some authors define lcm as 0 for all a, since 0 is the only common multiple of a and 0.
One way to find the least common denominator is to use prime factorization. Then multiply all of the prime factors, multiplying the factors that are common to both only once, to find the least common denominator. For example, when finding the GCF of \(180\) and \(162\), we start by listing the prime factorization of each number . The prime factorization of \(180\) is \(2\times2\times3\times3\times5\), and the prime factorization of \(162\) is \(2\times3\times3\times3\times3\).
Now look for the factors that are shared by both numbers. In this case, both numbers share one \(2\), and two \(3\)s, or \(2\times3\times3\). The result of \(2\times3\times3\) is \(18\), which is the GCF! This strategy is often more efficient when finding the GCF of really large numbers. You may also see the phrase least common multiple.
This generally refers to whole numbers, but the methods to find it are the same for both. Determining the least common denominator allows you convert the denominators to the same number so you can then add and subtract them. In order to add or subtract fractions with different denominators , you must first find a common denominator shared between them. In order to have the simplest fraction at the end, it is best to find not just a common denominator, but the least common denominator. This refers to the lowest multiple shared by each original denominator in the equation, or the smallest whole number that can be divided by each denominator. In mathematics, the least common multiple, also known as the lowest common multiple of two integers a and b, is the smallest positive integer that is divisible by both.
First, we need to find the lowest common denominator of our fractions. Read about the four methods below and choose the way you like the most. The least common multiple (L.C.M.) of two or more numbers is the smallest number which can be exactly divided by each of the given number. The lowest common multiple or LCM of two or more numbers is the smallest of all common multiples. Find the least common multiple (L.C.M) of 9 and 15 by using prime factorization method.
To find least common multiple by using prime factorization method is discussed here. The least common denominator of two fractions is the least common multiple of their denominators. For example, when finding the LCM, start by finding the prime factorization of each number . The prime factorization of \(20\) is \(2\times2\times5\), and the prime factorization of \(32\) is \(2\times2\times2\times2\times2\). Circle the factors that are in common and only count these once.
In the context of adding or subtracting fractions, the least common multiple is referred to as the least common denominator. On the DHB-E LCD instantaneous water heater, the water temperature is continuously electronically controlled by three sensors with 3i technology. This makes temperature fluctuations a thing of the past and ensures accurate temperature delivery up to the maximum output, making showering a delight every time. With the LCD of the DHB-E LCD comfort instantaneous water heater, accurate temperature settings are no longer a problem. The rotary selector quickly enables accurate temperature delivery between 20 and 60C. The easiest way to do it is to multiply any two or three of those numbers and see if the product is also a multiple of each of the other numbers.
Then divide that number by any small number to see if there's a lower common multiple. In this case you'll find that the lowest common multiple is the full product of 7, 8, 9 and 10, which is 5,040. To find the common denominators, we need multiples of 2 and 3.
Venn diagrams are drawn as overlapping circles. They are used to show common elements, or intersections, between 2 or more objects. Once the Venn diagram is completed you can find the LCM by finding the union of the elements shown in the diagram groups and multiplying them together. Find the least common denominator for a set of fractions by providing a list of denominators below. You have practiced adding and subtracting fractions with common denominators.
Now let's see what you need to do with fractions that have different denominators. As you know, there are times when we have to algebraically "adjust" how a number or an equation appears in order to proceed with our math work. We can use the greatest common factor and the least common multiple to do this. We are going to use a little tougher problem for adding fractions with different denominators to illustrate that you CAN do it.
Also, we will use Method #2 to find the least common denominator because it works best in almost every case. Above method is inefficient when larger numbers are involved. Therefore, we have to use prime factoring to obtain the common denominators. The lcm then can be found by multiplying all of the prime numbers in the diagram. To find the LCM of two or more numbers, we first find all the prime factors of the given numbers and write them one below the other. Take one factor from each common group of factors and find their product.
Multiply the product with other ungrouped factors. In our next video we show two more examples of how to use the column method to find the least common denominator of two fractions. Convert \frac[/latex] and \frac[/latex] to equivalent fractions with denominator 120[/latex], their LCD.
Convert \frac\text\frac[/latex] to equivalent fractions with denominator 12[/latex], their LCD. Let's approach this problem by listing the prime factors of both the numerator and the denominator. Listing the multiples is a great strategy when the numbers are fairly small. When numbers are large, such as \(38\) and \(42\), we should use the prime factorization approach. Start by listing the prime factorization of each number .
For larger numbers, it will not be realistic to make a list of factors or multiples to identify the GCF or LCM. For large numbers, it is most efficient to use the prime factorization technique. Divide the numbers by the factors of any of the three numbers. Nine and 3 cannot be divided by 2, so we'll just rewrite 9 and 3 here. Repeat this process until all of the numbers are reduced to 1.
Then, multiply all of the factors together to get the least common multiple. There are two ways of finding the lowest common multiple of two numbers. Identify the greatest common factor between both denominators. Once you have listed the factors of each denominator, circle all of the common factors.
The largest of the common factors is the greatest common factor that will be used to continue solving the problem.In our example, 8 and 12 share the factors 1, 2, and 4. While simply multiplying all of the denominators will get you a common denominator between the fractions, it does not always give you the LCD. Because 20 is the first shared multiple of 4 and 5, it must be the least common denominator for these two fractions. Because 28 is the first shared multiple of 4 and 7, it must be the least common denominator for these two fractions.
The Method of calculating the LCD is the same as the calculation least common multiple of fractions denominators. The least common denominator, also called lowest common denominator , of 21 and 18 is 126. You need to know the least common denominator of 21 and 18 if you want to add or subtract two fractions with 21 and 18 as denominators.
The least common denominator, also called lowest common denominator , of 18 and 21 is 126. You need to know the least common denominator of 18 and 21 if you want to add or subtract two fractions with 18 and 21 as denominators. We say that \frac\text\frac[/latex] are equivalent fractions and also that \frac\text\frac[/latex] are equivalent fractions. The denominator of the largest piece that covers both fractions is the least common denominator of the two fractions. So, the least common denominator of \frac[/latex] and \frac[/latex] is 6[/latex].